본 게시물은 University of Arizona의 Prof. J. Scott Tyo의 OPTI512R 강의를 보고 정리한 내용입니다.
전공자가 아닌 만큼 부정확한 정보가 포함되어 있을 수 있습니다.
부정확한 정보가 있다면 알려주시면 감사하겠습니다.
The Helmholtz equation
Engineering 분야에서 가장 유명한 Equation 중 하나를 꼽으라고 한다면 단연 Maxwell's equation일 것이다. Maxwell's equation은 Electric field와 Magnetic field에 대한 4개의 equation으로 Electrical Engineering에서 널리 사용된다. 이 Lecture에서는 Maxwell's equation을 Helmholtz equation이라는 특정한 형태로 줄이는 것에 대해 학습할 것이다.
다음과 같은 Maxwell's equation의 Time-harmonic form이 있다고 하자.
$$\triangledown \times \textbf{E} = -j\omega\mu \textbf{H} \\ \triangledown \times \textbf{H} = \textbf{J} + j\omega\epsilon \textbf{E} \\ \triangledown \cdot \textbf{E} = {\rho \over \epsilon} \\ \triangledown \cdot \textbf{H} = 0$$
여기서 $\textbf{E}$와 $\textbf{H}$는 각각 Electric field phasor와 Magnetic field phasor이고, $\mu$와 $\epsilon$은 각각 medium의 magnetic permeability와 electric permittivity이며, $j$는 current density, $\rho$는 charge density이다.
magnetic vector potential $\textbf{A}$와 scalar electric potential $\phi$는 다음의 definition과 함께 field를 계산하는 데 사용된다.
$$\textbf{H} = {1 \over \mu} \triangledown \times \textbf{A} \\ \textbf{E} -j\omega\textbf{A} - \triangledown \phi$$
이를 이용하여 위의 Maxwell's equation을 수정하면 아래와 같다.
$${1 \over \mu} \triangledown \times \triangledown \times \textbf{A} = \textbf{J} + j \omega \epsilon(-j\omega \textbf{A} - \triangledown \phi) \\ \triangledown(\triangledown \cdot \textbf{A}) - \triangledown^2\textbf{A} = \mu \textbf{J} + \omega^2 \mu \epsilon \textbf{A} - j\omega \mu \epsilon \triangledown \phi$$
Lorentz gauge condition에 의해 다음이 성립한다.
$$\triangledown \cdot \textbf{A} = -j\omega \mu \epsilon \phi$$
이를 위 수식에 적용하면 최종적으로 Helmholtz equation을 얻을 수 있다.
$$\triangledown ^2 \textbf{A} + k^2 \textbf{A} = -\mu \textbf{J}$$
Plane Wave Solutions
앞서 우리가 유도한 equation은 magnetic vector potential에 대한 Helmholtz equation(frequency domain wave equation)이다. 이 equation에 solution은 Plane wave, Spherical wave, fiber optic waveguid modes, antenna radiation pattern 등 다양한 solution이 존재한다. 이 Lecture에서 우리는 plane wave solution에 대해 알아볼 것이다.
$\rho =0$인 경우를 고려하고, $\textbf{J} = 0$인 region of space에 대한 Helmholtz equation의 solution을 살펴보도록 하자. 그 중 우리는 x-direction과 y-direction으로 일정하고, z의 function으로만 변화하는 solution에 대해 다룰것이다. 또한 current는 오직 x-direction으로만 흐른다고 가정할 것이다. 이러한 가정을 통해 $\textbf{J} = 0$인 region에서 helmholtz equation은 다음과 같이 정리된다.
$${d^2 A_x \over dz^2} + k^2 A_x = 0$$
이는 가장 기본적인 $2^{nd}$ order ODE(Ordinary Differential Equation)이고 다음과 같은 solution을 가진다.
$$A_x = A_{\pm} e^{\pm jkz}$$
free charge $\rho = 0일 때, electric potential $\phi = 0$ 이므로, 해당 electric field solution은 다음과 같은 형식을 가진다.
$$E_x = E_{\pm} e^{\pm jkz}$$
우리는 이것을 z-direction으로 propagate 되는 x-polarized plane wave라고 인식한다. 물론 우리는 같은 형태의 y-polarized plane wave도 가질 수 있다. 또한 paropagation이 z-direction에만 존재해야 할 필요도 없다. 일반적으로 $\hat{\textbf{k}}$ direction으로 propagation을 다음과 같이 표현한다.
$$\textbf{E}(\textbf{r}) = \textbf{E}_0 e^{\pm j \textbf{r} \cdot \textbf{k}}$$
여기서 $\textbf{K} = k_x \hat{\textbf{x}} + k_y \hat{\textbf{y}} + k_z \hat{\textbf{z}}$는 다음의 두 constrain에 종속된다.
$${k_x}^2 + {k_y}^2 +{k_z}^2 = \omega^2 \mu \epsilon$$
$$\textbf{k} \cdot \textbf{E}_0 = 0$$
이다. 첫 번째 constrain은 Helmholtz equation에서, 두 번째 constrain은 Maxwell equation에서 $\textbf{E}$의 divergence condition에서 비롯된다.
Exponential의 조사하면 다음을 통해 describe 되는 surface가 constant phase의 surface임을 알 수 있다.
$$\textbf{k} \cdot \textbf{r} = \it{const}$$
우리는 위 수식을 vector $\textbf{k}$에 normal한 plane equation으로 인식한다.
The Plane Wave Spectrum
앞으로는 optical field를 wave quantity $u(x,y,z)$로 표현하도록 하겠다. quantity $u$는 magnetic 혹인 electric filed이다. $u$는 scalar로 표현되고 있으며, 일반적으로 이것은 three spatial coordinate를 포함하는 function이다. 여기서는 Maxwell's equation의 simplification인 electromagnetic radiation과 diffraction을 고려하지 않을 것이다.
임의의 plane에서 field distribution을 안다고 할 때, 우리는 그 plane을 generality의 손실 없이 $z=0$ plane이라고 부를 수 있으며, 그 plane의 field는 $u(x,y)$이다. 수학적으로 우리는 Field를 Inverse Fourier Transform으로 다음과 같이 쓸 수 있다.
$$u(x,y) = \int \int^\infty_{-\infty} U(\xi, \eta) e^{j2\pi(\xi x + \eta y)}d\xi d\eta$$
$\xi$와 $\eta$는 spatial frequency vairable이다. 우리가 $u(x,y)$를 $U(\xi, \eta)$의 Inverser Fourier Transform으로 정의함으로써, 다음이 성립함을 알 수 있다.
$$U(\xi, \eta) = \mathcal{F} \{u(x,y)\} = \int\int^\infty_{-\infty} u(x,y) e^{-j2\pi(\xi x + \eta y)} dx dy$$
수식 $\textbf{E}(\textbf{r}) = \textbf{E}_0 e^{\pm j \textbf{r} \cdot \textbf{k}}$과 $u(x,y) = \int \int^\infty_{-\infty} U(\xi, \eta) e^{j2\pi(\xi x + \eta y)}d\xi d\eta$을 비교해보자.
$$\textbf{k} = {2\pi \over \lambda}(\gamma_x \hat{\textbf{x}} + \gamma_y \hat{\textbf{y}} + \gamma_z \hat{\textbf{z}})$$
$\textbf{k}$를 위와 같이 설정할 때, $\gamma_x$, $\gamma_y$ 및 $\gamma_z$는 아래 그림과 같이 Plane wave의 propagation direction을 indicate하는 direction cosine이다. $z=0$ plane에서 $\textbf{k} \cdot \textbf{r} = \it{const}$는 다음 값이 된다.
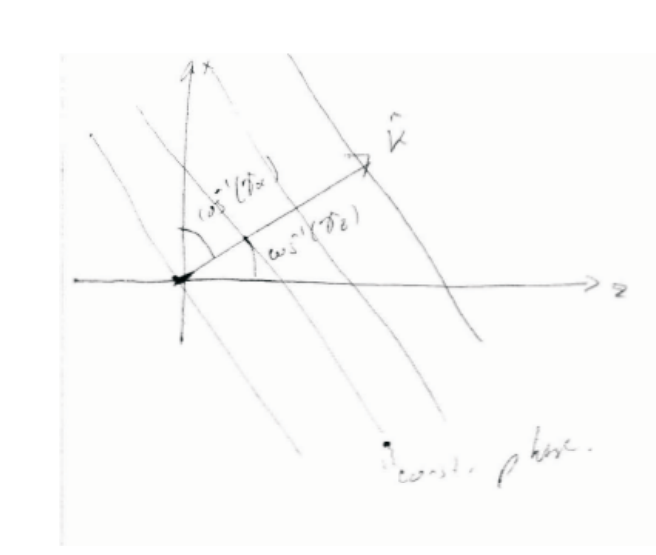
$$E_x = E_{\pm} e^{j2\pi({\gamma x \over \lambda} x + {\gamma y \over \lambda} y)}$$
최종 substitution $\xi = {\gamma_x \over \lambda}$와 $\eta = {\gamma_y \over \lambda}$를 수행하면, 우리는$u(x,y) = \int \int^\infty_{-\infty} U(\xi, \eta) e^{j2\pi(\xi x + \eta y)}d\xi d\eta$의 integrand가 단순히 direction cosine $\gamma_x = \lambda \xi$와 $\gamma_y = \lambda \eta$으로 propagating 하는 Complex amplitude $U(\xi, \eta)$의 Plane wave 임을 알 수 있다. ${k_x}^2 + {k_y}^2 +{k_z}^2 = \omega^2 \mu \epsilon$의 적용을 통해 다음을 알 수 있다.
$${\gamma_x}^2 + {\gamma_y}^2 + {\gamma_z}^2 = 1 \\ {\gamma_z} = \sqrt{1-({\gamma_x}^2 + {\gamma_y}^2)}$$
위 수식은 spatial frequency pair $(\xi, \eta)$에 해당하는 plane wave의 longitudinal propagation constant를 제공하기 때문에 매우 중요하다. ${\gamma_x}^2 + {\gamma_y}^2 <1$에 대해서 longitudinal propagation constant $\gamma_z$는 purely real이고, propagating plane wave mode $e^{\pm jk \gamma_z z}$를 갖는다. 그러나 ${\gamma_x}^2 + {\gamma_y}^2 >1$을 생성하는 $\xi$와 $\eta$의 value에 대해서는 $\gamma_z$는 puerly imaginary가 되고, plane wave는 $e^{-{2\pi \over \lambda} |\gamma_z|z}$evanescent(소멸)된다.
이제 real $\gamma_z$를 사용하는 propagating plane wave mode를 고려해보자, $z=0$일 때 plane wave mode는 다음과 같다.
$$U(\xi, \eta; z = 0) = U(\xi, \eta) e^{j2\pi(\xi x + \eta y)}$$
distance $\ell$만큼 propagating 된 후, plane wave는 다음과 같다.
$$U(\xi, \eta; z = \ell) = U(\xi, \eta) e^{jk \gamma_z \ell} \\ = U(\xi, \eta) e^{j2\pi (\xi x+ \eta y)}e^{jk\ell}\sqrt{1 - \lambda^2 (\xi ^2 + \eta^2)}$$
그러므로 우리는 distance $\ell$별로 propagation 후 spatial frequency $(\xi, \eta)$를 다음과 같이 계산할 수 있다.
$$H(\xi, \eta) = {U(\xi, \eta; z=\ell) \over U(\xi, \eta; z= 0)} = e^{jk\ell}\sqrt{1-\lambda^2(\xi^2 + \eta^2)}$$
1.Example 1 : A plane wave
$z=0$의 field가 $\textbf{k} = k_x \hat{\textbf{x}} + k_z \hat{\textbf{z}}$로 propagate하는 single plane wave로 구성된 경우를 생각해보자. 이 경우 $z=0$ plane의 field는 다음과 같다.
$$u(x,y, z=0) = u_0 e^{jk_x x} = u_0 e^{j2\pi {\gamma x \over \lambda}x}$$
위 수식에 Fourier Transform을 적용하면 다음과 같은 결과를 얻는다.
$$U(\xi, \eta) = u_0 \delta(\xi - {\gamma_x \over \lambda}) \delta(\eta)$$
이 경우 $z=0$ field의 plane wave spectrum은 $\delta-$function이므로 의미가 있고, Spectrum에는 $\gamma_x = \lambda \xi$및 $\gamma_y = 0$으로 propagating 되는 plane wave하나만 있다.
2. Example 2 : A Gaussian Beam
이제 $z=0$ plane에 있는 field가 다음과 같이 설명된다고 가정하자.
$$u(x,y, z= 0) = Gaus({x \over b})$$
이는 y-direction으로 constant 한 one-dimensional Gaussian field distribution을 describe 한다. Fourier Transform을 적용하여 Gaussian Beam의 Plane wave spectrum을 다음과 같이 결정한다.
$$U(\xi, \eta) = \mathcal{F}\{u(x,y,z=0)\} \\ = bGaus(b\xi)\delta(\eta)\\ = bGaus(b{\gamma_x \over \lambda})\delta(\eta)$$
위 수식은 Gaussian Beam이 모두 약간 다른 direction으로 이동하는 plane wave의 spectrum으로 구성되었음을 알려준다. 이 plane wave packet은 constant b에 의해 결정된다. $b \over \lambda$가 클 때, $(x,y)-$ plane 상의 Beam은 wavelength에 비해 크고, plane wave spectrum은 상당히 narrow 하다. 그러나 $b\ over lambda$가 작을 때, Gaussian beam을 form 하기 위해 합산되는 Wide range propagating plane wave를 얻게 된다.
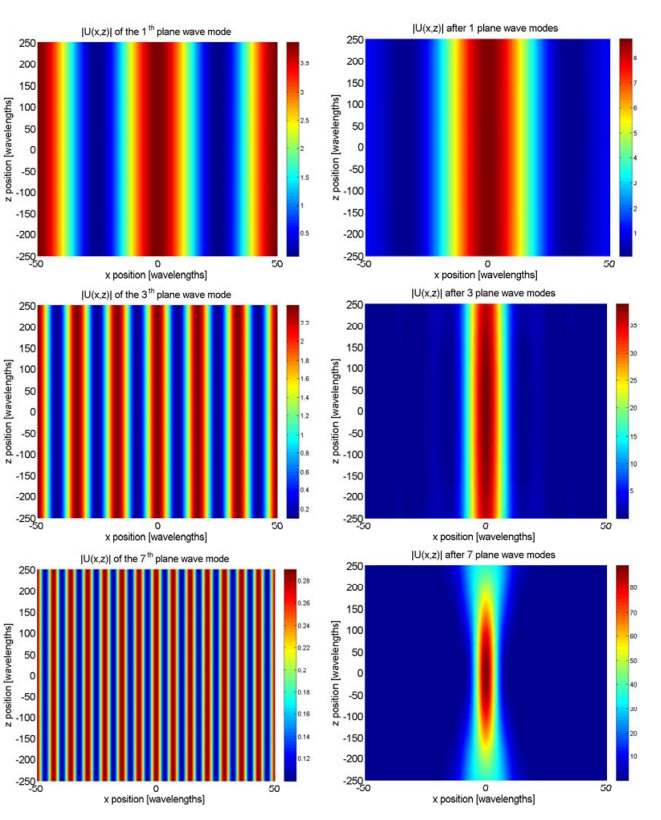
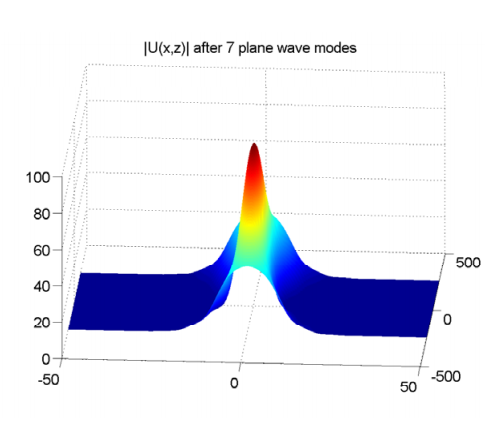
이것이 어떻게 동작하는지 알아보기 위해, Discrete plane wave mode(Gaussian의 DFT)를 추가하여 Gaussian Beam을 형성한다. 아래 그림은 Fourier Transform approximation에 점점 더 많은 mode가 추가됨에 따른 Gaussian Beam의 발전을 보여준다.
처음에는 0번째, 1번째 mode만이 존재하는 경우 field는 transverse direction에서는 oscillatory 하고, longitudinal direciton으로는 non-decaying 한다. 그러나 7번째 mode가 추가될 때쯤이 되면, Gaussian Beam의 특성이 명백해진다. Wavefront는 기본적으로 curved 하며, Beam waist에서 Clear Maximum Amplitude를 가진다. 이는 아래 그림에서 더 명확히 확인할 수 있다.
3. Example 3 : Evanescent Plane Wave
$z=0$ plane에 $\xi_0 > {1 \over \lambda}$를 만족하는 다음과 같은 field가 주어진다고 가정하자.
$$u(x,y) = \cos(2\pi \xi_0 x)$$
Example 1에서와 마찬가지로 plane wave spectrum은 다음과 같이 discrete 하다.
$$U(\xi, \eta) = {u_0 \over 2}(\delta(\xi - \xi_0) + \delta(\xi + \xi_0))\delta(\eta)$$
하지만 이 spatial frequency에서는 다음이 성립한다.
$$\gamma_z = \sqrt{1- \lambda^2 {\xi_0}^2} = j|\gamma_z|$$
Propagation constant가 purely imaginary이기 때문에, total field는 다음과 같은 exponential 하게 falling 하는 evanescent field로 쓸 수 있다.
$$ u_{tot}(x,y,z) = \cos(2\pi \xi_0 x) e^{-\sqrt{\lambda^2 {\xi_0}^2 -1}}$$
이러한 방식으로 우리는 field의 모든 high spatial frequency가 evanescent plane wave에 해당되고, propagating 과정에서 high spaital frequency information의 loss가 발생한다는 것을 알 수 있다.
4. Using The Plnae Wave Spectrum To Propagate Fields
이제 우리는 Gaussian Beam에 대한 Plane wave specturm을 얻었다. 이 plane wave를 사용하여 Beam waist에서 멀어져 가는 Gaussian propagation을 describe 할 수 있는지 알아보자. Beam의 spectrum은 다음과 같이 주어진다.
$$U(\xi, \eta) = {b_0}^2 e^{-\pi {b_0}^2 (\xi^2 + \eta^2)}$$
$z=0$ plane에서의 total field는 다음과 같이 주어진다.
$$u(x,y,z=0) = \mathcal{F}^{-1} \{ U(\xi, \eta)\}$$
$z=0$에서 $z=z$로 propagating 할 때, 각 plane wave에 다음과 같은 transfer function이 multiply 된다.
$$H(\xi, \eta) = e^{jkz \sqrt{1-\lambda^2(\xi^2 + \eta^2)}}$$
따라서 $b_0 >> \lambda$ 인 경우 다음이 성립한다.
$$U(\xi, \eta; z) = U(\xi, \eta; z=0) e^{jkz\sqrt{1-\lambda^2(\xi^2 + \eta^2)}} \\ \approx {b_0}^2 e^{-\pi {b_0}^2(\xi^2 + \eta^2) + j{2\pi z \over \lambda}(1- {\lambda^2 \over 2}(\xi^2 + \eta^2))}$$
이 approximation은 Gaussian envelope가 rapidly fall off 하고, $\lambda^2(\xi^2 + \eta^2) << 1$이기 때문에 valid 하다. 우리는 이를 정리하여 다음과 같이 정리할 수 있다.
$$U(\xi, \eta; z) = {b_0}^2 e^{jkz} e^{-\pi[{b_0}^2 + j\lambda z](\xi^2 + \eta^2)}$$
spatial domain의 field를 계산하기 위해 우리는 위 수식에 Inverse Fourier Transform을 적용하여 다음을 얻을 수 있다.
$$u(x,y,z) = \mathcal{F}^{-1} \{U(\xi, \eta;z)\} \\ = e^{jkz} {{b_0}^2 \over{b_0}^2 + j\lambda z} e^{-\pi {{x^2 + y^2} \over {b_0}^2 + j\lambda z}}\\ = e^{jkz} {{b_0}^2 \over{b_0}^2 + j\lambda z} e^{-\pi {{b_0}^2 \over {b_0}^4 + (\lambda z)^2}(x^2 + y^2)} e^{j{\pi\lambda z \over {{b_0}^4} + (\lambda z)^2}(x^2 + y^2)}$$
첫 번째 항은 Gaussian profile $Gaus({r \over b(z)})$이다. 이때 $b(z)$는 다음과 같다.
$$b(z) = b_0 \sqrt{1 + ({\lambda z \over {b_0}^2})^2}$$
두 번째 항은 $e^{j({\pi \over \lambda R(z)})(x^2 + y^2)}$이고, $R(z)$는 다음과 같다.
$$R(z) = z[({{b_0}^2 \over \lambda z}^2) + 1]$$
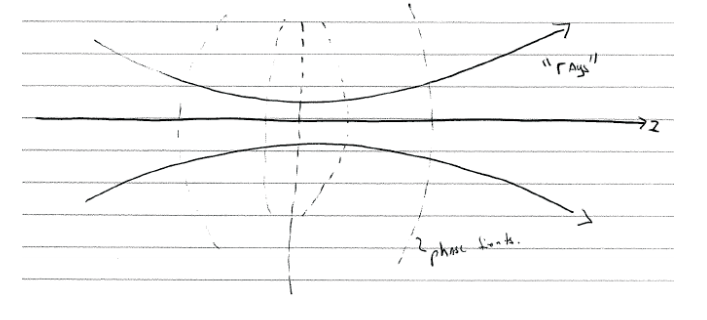
다음 몇 개의 lecture들을 통해 이 항이 Curvature $R(z)$ radius를 가지는 spherical wave에 대한 quadratic phase approximation에 해당함을 알게 될 것이다. $R(z) > 0 (z > 0)$일 때, wave가 diverging 하고, $R(z) < 0(z < 0)$일 때, wave 가 converging 하고 있다. Gaussian Beam의 wave front와 "ray"는 아래 그림에 표현되어있다.
'Optics > 이론' 카테고리의 다른 글
| Lec 17. Fresnel Diffraction (1) | 2021.08.24 |
|---|---|
| Lec 16. Fraunhofer Diffraction (0) | 2021.08.23 |
| Lec 14. Discrete Convolution (0) | 2021.08.16 |
| Lec 13. Discrete Fourier Transform (0) | 2021.08.16 |
| Lec 12. Sampling & Reconstruction (2) | 2021.08.14 |